심슨의 역설
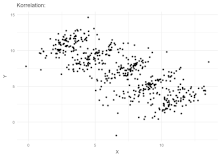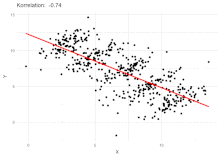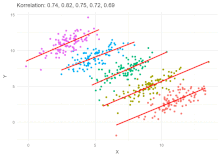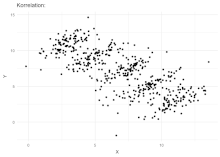
심슨의 역설(Simpson's paradox)은 데이터의 세부 그룹별로 일정한 추세나 경향성이 나타나지만, 전체적으로 보면 그 추세가 사라지거나 반대 방향의 경향성을 나타내는 현상을 의미한다. 이 현상은 사회과학이나 의학 통계 연구에서 종종 발생한다.[1][2][3] 심슨의 역설은 통계의 함정이 유발할 수 있는 잘못된 결과를 설명하는 데 쓰이기도 한다.

에드워드 심슨이 1951년 처음으로 이 현상을 설명한 것으로 알려져 있으나,[4] 1899년 칼 피어슨[5], 1903년 우드니 율[6]이 유사한 현상에 관해 설명한 적이 있다. "심슨의 역설"이라는 이름은 1972년 콜린 블리스라는 학자가 사용하였다.[7]
사례 편집
신장결석 치료법 편집
심슨의 역설을 보여주는 하나의 사례는 신장결석 치료법에 관한 의학 연구이다.[8][9] 아래의 표는 작은 크기의 신장결석과 큰 크기의 신장결석에 대해 두 가지 치료법을 적용한 결과 성공률을 나타낸 표이다.
치료법 결석 크기
|
치료법 A | 치료법 B |
|---|---|---|
| 작은 결석 | 그룹 1 93% (81/87) |
그룹 2 87% (234/270) |
| 큰 결석 | 그룹 3 73% (192/263) |
그룹 4 69% (55/80) |
| 모두 | 78% (273/350) | 83% (289/350) |
작은 결석과 큰 결석 모두에서 치료법 A의 성공률이 높게 나왔지만, 결석의 크기를 구분하지 않고 합친 경우에는 치료법 B의 성공률이 높은 결과가 나왔다. 이 사례에서는 결석의 크기라는 숨겨진 변수 또는 혼재변수가 각 치료법의 성공률에 영향을 미친 경우에 해당된다. 결석의 크기에 따라 성공률 자체가 달라지며, 결석의 크기 등과 같은 환자의 특성에 따라 선택하는 치료법이 달라진다는 것[9]이 심슨의 역설 현상을 낳게 하였다.
벡터 해석 편집
심슨의 역설은 2차원 벡터의 기울기를 비교하는 방법으로 보일 수 있다.[10] B1은 L1보다 가파르고, B2 역시 L2보다 가파른 경우에도 B1+B2는 L1+L2보다 완만한 기울기를 가질 수 있다.
각주 편집
- ↑ Clifford H. Wagner (February 1982). “Simpson's Paradox in Real Life”. 《The American Statistician》 36 (1): 46–48. doi:10.2307/2684093. JSTOR 2684093.
- ↑ Holt, G. B. (2016). Potential Simpson's paradox in multicenter study of intraperitoneal chemotherapy for ovarian cancer. Journal of Clinical Oncology, 34(9), 1016–1016.
- ↑ Franks, Alexander; Airoldi, Edoardo; Slavov, Nikolai (2017). “Post-transcriptional regulation across human tissues”. 《PLOS Computational Biology》 13 (5): e1005535. arXiv:1506.00219. doi:10.1371/journal.pcbi.1005535. ISSN 1553-7358. PMC 5440056. PMID 28481885.
- ↑ Simpson, Edward H. (1951). “The Interpretation of Interaction in Contingency Tables”. 《Journal of the Royal Statistical Society, Series B》 13: 238–241.
- ↑ Pearson, Karl; Lee, Alice; Bramley-Moore, Lesley (1899). “Genetic (reproductive) selection: Inheritance of fertility in man, and of fecundity in thoroughbred racehorses”. 《Philosophical Transactions of the Royal Society A》 192: 257–330. doi:10.1098/rsta.1899.0006.
- ↑ G. U. Yule (1903). “Notes on the Theory of Association of Attributes in Statistics”. 《Biometrika》 2 (2): 121–134. doi:10.1093/biomet/2.2.121.
- ↑ Colin R. Blyth (June 1972). “On Simpson's Paradox and the Sure-Thing Principle”. 《Journal of the American Statistical Association》 67 (338): 364–366. doi:10.2307/2284382. JSTOR 2284382.
- ↑ C. R. Charig; D. R. Webb; S. R. Payne; J. E. Wickham (1986년 3월 29일). “Comparison of treatment of renal calculi by open surgery, percutaneous nephrolithotomy, and extracorporeal shockwave lithotripsy”. 《British Medical Journal》 292 (6524): 879–882. doi:10.1136/bmj.292.6524.879. PMC 1339981. PMID 3083922.
- ↑ 가 나 Steven A. Julious; Mark A. Mullee (1994년 12월 3일). “Confounding and Simpson's paradox”. 《BMJ》 309 (6967): 1480–1481. doi:10.1136/bmj.309.6967.1480. PMC 2541623. PMID 7804052.
- ↑ Kocik Jerzy (2001). “Proofs without Words: Simpson's Paradox” (PDF). 《Mathematics Magazine》 74 (5): 399. doi:10.2307/2691038. JSTOR 2691038.
외부 링크 편집
- Simpson's Paradox at the Stanford Encyclopedia of Philosophy, by Jan Sprenger and Naftali Weinberger.
- How statistics can be misleading – Mark Liddell – TED-Ed 비디오 강좌.
- 유디 펄, "Understanding Simpson’s Paradox" (PDF)
- Simpson's Paradox, a short article by Alexander Bogomolny on the vector intepretation of Simpson's paradox
- The Wall Street Journal column "The Numbers Guy" for December 2, 2009 dealt with recent instances of Simpson's paradox in the news. Notably a Simpson's paradox in the comparison of unemployment rates of the 2009 recession with the 1983 recession.
- At the Plate, a Statistical Puzzler: Understanding Simpson's Paradox by Arthur Smith, August 20, 2010
- Simpson's Paradox, a video by Henry Reich of MinutePhysics